Solution of the Week #530 - Rhombus Stack

Opposite sides of a rhombus are parallel and the same length, therefore the length and orientation of the lower left side of the square are identical to the right hand slope of the triangle with base 18. Similarly the lower right side of the square has the same length and orientation as the left slope of the 38 base triangle. We can now dispense with the rest of the diagram and only look at those two triangles, armed with the knowledge that the angle between them is 90 degrees. This also means that the length of the other two triangle bases was irrelevant, so long as they produced valid triangles.

Dropping perpendicular bisectors from each we get two congruent right angled triangle with legs 9 and 19.

Since the area we seek is simply x^2, we can use Pythagoras to find that the area of the original square is 9^2+19^2, which is equal to 442.

Solution of the Week #529 - Rectangles in a Quarter Circle

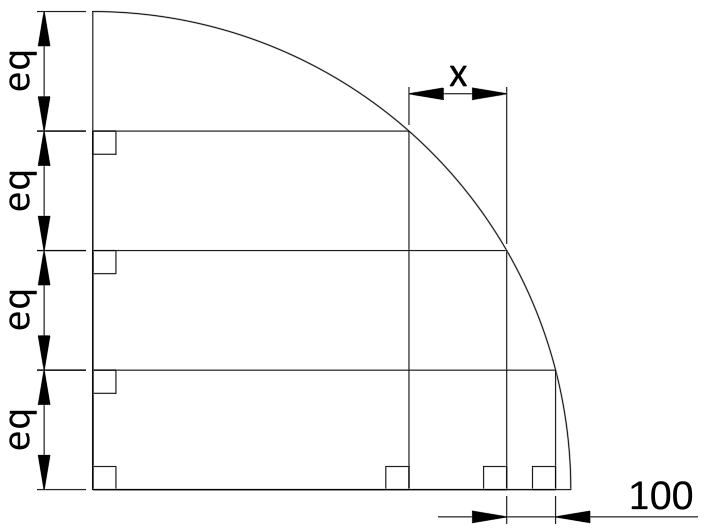
Let’s call the equal heights h. The radius of the quarter circle and therefore also the diagonal of each of the three main rectangles is 4h. The horizontal length of each of those rectangles, let’s call them k,l,m can be found using Pythagoras:

k^2=(4h)2-h^2=15h^2

l^2=(4h)2-(2h)^2=12h^2

m^2=(4h)2-(3h)^2=7h^2

k=h*sqrt(15)

l=h*sqrt(12)

m=h*sqrt(7)

Now 100 and x are simply the difference between those lengths:

100=k-l=h*(sqrt(15)-sqrt(12))

x=l-m=h*(sqrt(12)-sqrt(7))

If we divide one equation by the other we can eliminate h:

x/100 = (sqrt(12)-sqrt(7))/(sqrt(15)-sqrt(12))

x/100 = 2.0014

x = 200.14, or 200 to the nearest integer.

Why should (sqrt(12)-sqrt(7))/(sqrt(15)-sqrt(12)) be so close to 2?

None of 7 12 or 15 is a square number so we can’t reduce them any further. When we factorise them nothing useful happens. However if we multiply each of them by 24, the above ratio will be preserved, and although 168, 288 and 360 still aren’t square numbers, the numbers just above them, 169, 289 and 361 all are (squares of 13, 17 and 19 respectively).

The value of (sqrt(289)-sqrt(169))/(sqrt(361)-sqrt(289)) is precisely 2, so it should come as no surprise that (sqrt(288)-sqrt(168))/(sqrt(360)-sqrt(288)) is so close to 2, and THAT ratio is precisely the same as the one in the question.

Why is it that when we multiply each of the numbers (7,12,15) by 24 we get a number one less than a square? Is that just a coincidence? Is it always possible so multiply a set of numbers by a particular factor so that the products are all one less than a square? These are interesting questions.

Solution of the Week #527 - Allesley Park part 3

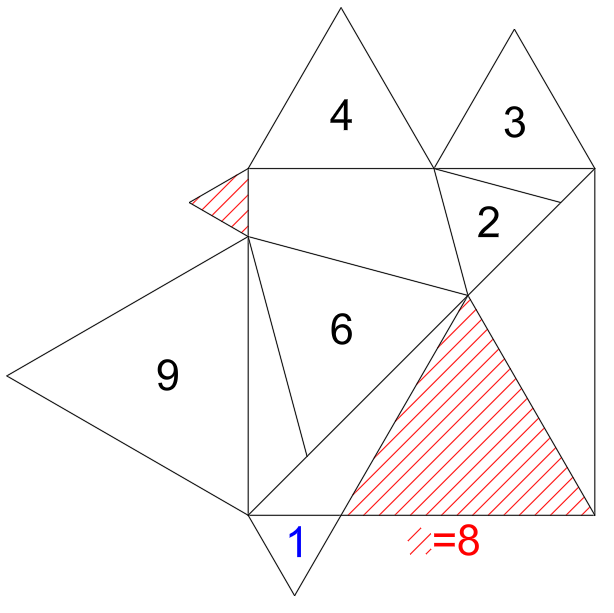
A Linean Cycle is not possible for a cube.

If we mark the doubly traversed edges in red, there are two distnict ways to colour the graph, and since at each vertex we arrive and depart, and since the red edges are responsible for net zero arrivals-departures, so must the black edges. Without loss of generality we can add directionality to the black edges such that they form a loop. A valid path must go red – black – red – black etc, since not doing so would result in a red edge being traversed consecutively. With one of the arrangements of red edges, the black edges form two smaller loops and so we must try them both going the same way, or going different ways.

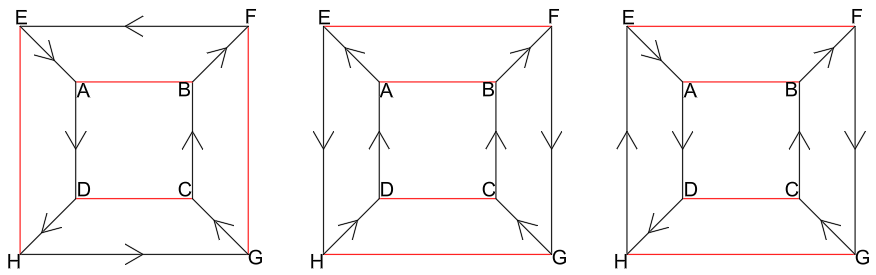
In the first configuration, if we start with AB we go ABFGCDHE(AB…) completing a loop before all edges have been traversed.

Likewise in the second: ABFEHGCD(AB…), and the third: ABFE(AB…)

I’ll leave it for the reader to make a Linean cycle for the tetrahedron, dodecahedron or icosahedron.

Solution of the Week #526 - Allesley Park part 2

It is possible to run four of the repeated segments bidirectionally, and only incur penalty points on two paths. The network of paths is topologically equivalent to the edges of a truncated tetrahedron, and the same-direction repeated edges will be opposite edges of one of the hexagonal faces. That being the case the ‘cheapest’ edges to take the penalty points for are BL and HI, incurring only 5 penalty points in total.

The way to achieve this is A-C-B-L-J-K-E-D-C-A-B-L-K-J-H-I-G-F-D-E-F-G-H-I-A (or its reverse, or beginning at any other point in the cycle).  

Solution of the Week #525 - Allesley Park part 1

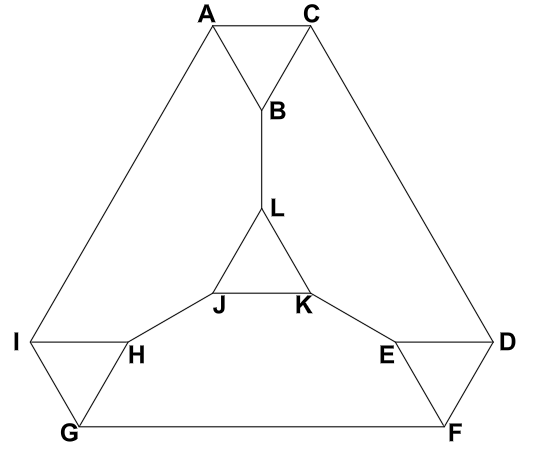
You might be aware that to complete a route and return to where you started you need to visit each junction point an even number of times. However, each of the 12 junction points on the map has an odd number of paths coming from them, so we need to repeat some of the paths to change all of the odds nodes to even. This can be done in several ways but the one that has the least extra mileage is to repeat AC, BL, JK, HI, FG and DE.

So the best we can do is to run all the paths and return to where we started in 2.24 miles.

Solution of the Week #524 - Four Areas

We’ll start by drawing a line dividing the 19 region into two. We’ll call one ‘a’ and by definition the other will be 19-a.

Now, we don’t know where the line from the apex hits the base, but we can say that the ratio of the left half to the right half is the same whether we are talking about the full height triangle or just the 6 and 19-a regions. In other words:

(19-a)/6 = 27/(x+6)

Let’s manipulate to find an expressions for a:

19-a = 162/(x+6)

a = 19-162/(x+6)

If we were to rotate the figure we can do exactly the same but with the 8 and a regions along the base:

a/8 = 25/(x+8)

a = 200/(x+8)

Now we have an equation involving both expressions for a:

19-162/(x+6) = 200/(x+8)

19(x+8)-162*(x+8)/(x+6) = 200

19x+152-200 = 162*(x+8)/(x+6)

(19x-48)*(x+6) = 162*(x+8)

19x^2-48x+114x-288 = 162x+1296

19x^2-96x-1584=0

Using the quadratic formula the positive value of the missing area x is 12.

Solution of the Week #523 - Equal Sequences

Part 1: [1,4,3] and [3,2,3]

1+4+16 = 3+6+12 = 21

Part 2: [1,6,4] and [37,2,3]

1+6+36+216 = 37+74+148 = 259

Solution of the Week #522 - Geometric Sequence

Unlike before, we cannot simply take the mean of each sequence and make them equal. The geometric means of the sequences would be equal, but we aren’t given the product of each sequence so that doesn’t help. As before we don’t know how many terms in the sequence, but we do know it must be of the form 6m+1.

The formula for the sum of a (finite) geometric sequence is:

S = a*(r^n-1)/(r-1) (r not equal to 1)

We are told our initial term is 1, so the a can be omitted from the formula.

For the main sequence the common ratio is simply r, and the number of terms n is 6m+1:

127 = (r^(6m+1)-1)/(r-1)

127(r-1) = r^6m*r – 1; let’s call r^6m = k

127r-126 = kr

k = 127-126/r

For our second sequence there are 3m+1 terms and the common ratio is r^2:

85 = (r^2(3m+1)-1)/(r^2-1)

85(r^2-1) = r^(6m+2)-1

85r^2-84 = kr^2

Let’s plug in the expression for k we found above:

85r^2-84 = (127-126/r)r^2

85r^2-84 = 127r^2-126r

42r^2 – 126r + 84 = 0

Solve the quadratic to find r = 1 or 2.

The formula for the sum of a geometric sequence specifies that r should not be exactly 1, but we’ll try it anyway if only to eliminate it as a possibility. The full sequence would just be 127 instances of the number 1. The second sequence would have 64 instances of 1 and therefore total 64, but this is not the case, so r=1 is not a valid solution.

If r = 2, the sequence goes 1,2,4,8 etc. The first 7 terms total 127. But if we want to be strictly systematic we know that k=127-126/2, so k=64. r^6m = k, so 2^6m = 64. m is therefore equal to 1. n, the number of terms in the sequence, is 6m+1, and therefore equal to 7.

Now to check the second sequence, 1+4+16+64=85, which is correct.

Finally to answer the question of the sum of the third sequence:

1+8+64 = 73.

Solution of the Week #521 - Arithmetic Sequence

We don’t know how many terms in the whole sequence but if all three sequences start and end at the same point, there must be both an odd number of terms and one more than a multiple of three terms. So there must be 6m+1 terms.

S is the sum of all 6m+1 terms.

88 is the sum of 3m+1 terms.

60 is the sum of 2m+1 terms.

All three sequences will have the same average since they are arithmetic sequences beginning and ending on the same terms, therefore:

S/(6m+1)=88/(3m+1)=60/(2m+1)

Taking just the last two terms and cross-multiplying:

88(2m+1)=60(3m+1)

176m+88=180m+60

4m=28

m=7

There are therefore 6*7+1=43 terms in the whole sequence.

Now just taking the first two terms and using the newly discovered value for m:

S*22=88*43

S=172

In all three sequences the average of the sequence will be 4. Therefore the middle (22nd)term of the full sequence will be 4.

If the first term is 0, and it needs 21 steps to get from there to the 22nd term, the common difference will be 4/21.

Solution of the Week #520 - Irregular Octagon

We can do this the easy way or the hard way.

The hard way involves setting up and solving a series of equations involving a,b,s and r in the above figure. And then noting that the area of the octagon is (2r)^2-2ab.

The easy way is to note that by drawing lines from each vertex to the centre, the octagon can be dissected into eight triangles, each of base s and height r. So the area is simply 4rs, which given s=17 and r=19 gives an area of 1292.

I recently used the fact that there are two ways to calculate the area to provide a proof of Pythagoras Theorem.

Solution of the Week #519 - Word Pairs

COPPER, CHOPPER, HOP, HOPE, FATTEN, FLATTEN, SPIT, SPLIT, RUSE, ROUSE

The extra letters spell HELLO.

Solution of the Week #518 - Four Rectangles

In the general case of two rectangles overlaid such that they have one corner in common and each has a corner coinciding with the side of the other rectangle, it will produce a pair of similar triangles as below. From this we can tell that C/A=B/D. Cross-multiplying shows that AB=CD, in other words the two rectangles have the same area. So in the original figure, all four rectangles have the same area. We can’t know the exact length and width of the red and blue rectangles, but since the green and magenta rectangles also share an edge, that gives us enough information to solve the puzzle. If we call the height of the magenta rectangle ‘x’, then the height of the green rectangle is x-14.

36*(x-14)=27x

9x=36*14

x=56

Solution of the Week #517 - Friday April 18th

If the days of the week are arranged in alphabetical order, Friday is first and Wednesday is last. For the months of the year April is first and September is last, and for the dates when written out in words, eighteenth is first and twenty third is last.

Solution of the Week #516 - Two Pairs of Circles

The answer is that the radius of the incircle is 7. I only really know that because I already knew that when I constructed the puzzle. I have an extremely messy and complicated way to find it only given the information in the question, which I won’t bore you with, but if you found it in a neat way please do let me know.

Edit: see comments on the puzzle page, neat solutions to be found there.

Solution of the Week #515 - Three Circles in a Trapezoid

Following the previous puzzles and the relationships between lengths of a right trapezium with an inscribed circle, if we were to follow that through to equations I believe we would end up with quartic equations awkward to work with.

More practically in this instance we can just use trial and error, and very quickly find that each trapezoid as we descend the figure is 50% bigger than the one above. So the horizontal lines measure 40, 60, 90 and 135. The sloping lines will then be 51, 78 and 117. And the vertical lines will be 48, 72 and 108, giving the overall height of 228.

Solution of the Week #514 - Two More Circles in Trapezoids

If we call the length of the middle horizontal x, we can use the formula for the radius of the incircles as before to determine the height of each trapezoid in terms of x.

Since we are told the angle on the right is a right angle, we can find two similar triangles above and below it (in fact they are congruent, but we don’t need to assume that for our solution).

So (x-5)(x-12)=10x/(x+5) * 24x/(x=12)

(x+5)(x-5)(x+12)(x-12)=240x^2

(x^2-25)(x^2-144)=240x^2

Let’s make a substitution and call x^2 y

(y-25)(y-144)=240y

y^2-409y+3600=0

y=9 or 400

x therefore is +/-3 or +/-20

In the diagram, it is clearly bigger than 12, so must be equal to 20.

Plugging this value into the height expressions above gives the overall height as 8+15=23.

Solution of the Week #513 - Two Circles in a Trapezoid

The trivial answer is if A=B=C=D, wherein A/B = 1.

To find the other solution, first consider that the top part of the shape is similar, in the mathematical sense, to the bottom part. Let’s say that the top horizontal has length 1, then the middle horizontal has some unknown factor x, and the bottom horizontal will therefore be x^2.

Next let’s look at the properties of a right trapezoid with a circle inscribed within it.

Splitting the lengths at the tangent points we call the radius of the circle R and the other two lengths S and T, forming a right angled triangle to combine them.

Using Pythagoras, (T+S)^2-(T-S)^2=(2R)^2, therefore ST=R^2.

Now let’s define S as U-R and T as V-R.

(U-R)(V-R)=R^2

UV-R(U+V)+R^2=R^2

UV=R(U+V)

R=UV/(U+V)

Therefore we can find the radius of a circle within a right trapezoid as simply the product of the top and bottom sides divided by their sum. The height of the trapezoid will be twice that.

And for completeness, the sloping side will be the sum of the top and bottom sides, less the height of the trapezium.

Let’s now look at the top trapezoid in the puzzle, where we have defined the top edge to be 1 and the bottom edge to be x.

The left side will be 2x/(1+x). That denominator is awkward, so let’s scale everything up by (1+x). We can do that because ultimately it is a ratio between sides that we are seeking.

Now remember everything in the bottom trapezoid is the same as the top one, but scaled up by x. We can therefore find expressions for A, B, C and D in terms of x:

A=2x^2

B=x+1

C=2x

D=x^3+x

We are told that A+C=B+D

2x^2+2x=x^3+2x+1

x^3-2x^2+1=0

We know that one solution (the trivial solution) is that x=1, which leaves the quadratic x^2-x-1=0. This has one positive solution: (1+sqrt(5))/2, or the golden ratio.

Finally, plugging this into the expressions for A and B gives a ratio for A/B of exactly 2.

So the solution is A/B = 2.

If we rescale back to letting B=1, A=2, C will be sqrt(5)-1 and D is sqrt(5). The diagonal line will have a gradient of -2. And of course the middle horizontal will be equal to the golden ratio.

Solution of the Week #513 - Two Circles in a trapezoid

The trivial answer is if A=B=C=D, wherein A/B = 1.

I couldn’t find an elegant way to get to the other solution, so I won’t attempt to present one. I’ll just tell you that the solution is A/B = 2.

If we let B=1, A=2, C will be sqrt(5)-1 and D is sqrt(5). The diagonal line will have a gradient of -2. The middle horizontal will be equal to the golden ratio.

Solution of the Week #512 - 4x4x4 Cube

The argument for the 3x3x3 version hinges on the fact that the central cube will have 6 newly cut faces, and therefore will require 6 slices to form, regardless of rearrangement. For the 4x4x4 cube, there are 8 inner cubes, each of which need 6 slices directly acting on them. This can be done as long as the first cut in each direction is the middle one, and then the two halves can be lined up to simultaneously perform the other cuts in that direction. So perhaps counter-intuitively, a 4x4x4 cube also only needs 6 slices.