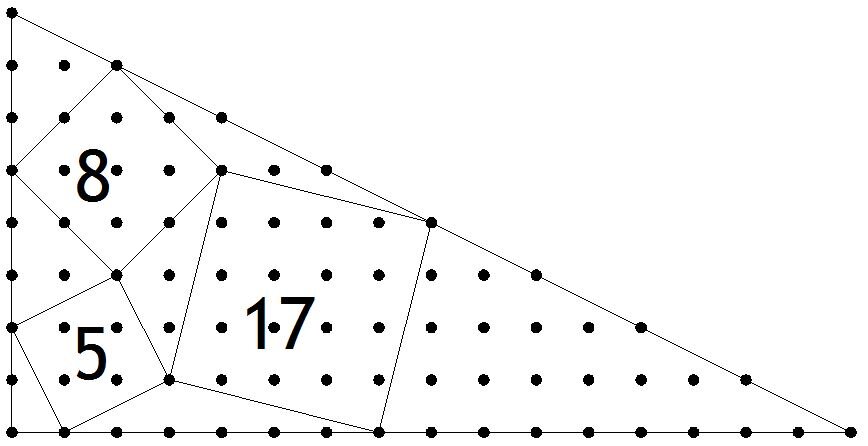
I don’t know if having a related puzzle last week is a help or a hindrance, as my approach to solving, for what it’s worth, is not at all similar to last week.
I notice that the given areas are each the sum of two squares (5=4+1, 8=4+4, 17=16+1). In general, if the area is a^2+b^2, then it’s at least possible that each side of the square is a units in one direction, and b units in a perpendicular direction.
On a hunch I hopefully set out lattice points of a coordinate system, and sure enough, each of the corners of each of the squares lies exactly on a lattice point. It is then straightforward to work out what the coordinates of the triangle corners are and then calculate the area of the triangle is 64.